Beschreibung01 Quadratur des Kreises E-8.svg	Deutsch: Quadratur des Kreises, Näherungskonstruktion mithilfe ${\displaystyle {\sqrt {2}}}$ English: Squaring the circle, approximate construction with help ${\displaystyle {\sqrt {2}}}$
Datum	27. Dezember 2019
Quelle	Eigenes Werk
Urheber	Petrus3743
SVG‑Erstellung InfoField	Der SVG-Code ist valide.   Dieses Diagramm wurde von Petrus3743 mit GeoGebra erstellt.   This SVG trigonometry uses the path text method.

Die Näherung von ${\displaystyle {\frac {a}{2}}}$ beruht auf den Wert

${\displaystyle {\overline {KL}}={\frac {a}{2}}=1-{\frac {{\sqrt {2}}\left(2-{\sqrt {2}}\right)\left(2-{\sqrt {2}}+{\sqrt {{\frac {1}{2}}+{\sqrt {2}}}}\right)}{-11+11{\sqrt {2}}+5{\sqrt {1+2{\sqrt {2}}}}}}\approx r\cdot \ 0{,}886\;226\;932\;777\;110\;[\mathrm {LE} ]}$

Die Verwendung der Quadratwurzel aus 2 = ${\displaystyle |FJ|}$ liefert eine Näherung von ${\displaystyle \pi ,}$ die bereits im Jahr 1913 in der Konstruktion von Jakob de Gelder mit dem Zu Chongzhi-Bruch ${\displaystyle {\frac {355}{113}}}$ nahezu erreicht wurde.

• Ergebnis der folgenden Konstruktion

${\displaystyle 3{,}141\;592\;{\color {red}\;705\;\ldots }}$

• Ergebnis mit Zu Chongzhi-Bruch ${\displaystyle {\frac {355}{113}}}$

${\displaystyle 3.141\;592\;{\color {red}\;920\;\ldots }}$

• Kreiszahl ${\displaystyle \pi }$

${\displaystyle 3{,}141\;592\;653\ldots }$

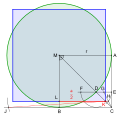
1. Ziehe einen Kreis mit beliebigem Radius ${\displaystyle r}$ um seinen Mittelpunkt ${\displaystyle M.}$
2. Zeichne das Quadrat ${\displaystyle MBCA}$ mit ${\displaystyle r}$ als Seitenlänge und verlängere die Strecke ${\displaystyle {\overline {CB}}}$ über ${\displaystyle B}$ hinaus.
3. Ziehe die Diagonale ${\displaystyle {\overline {MC}},}$ ergibt Schnittpunkt ${\displaystyle D.}$
4. Zeichne eine Parallele zu ${\displaystyle {\overline {BC}}}$ durch ${\displaystyle D}$ bis ${\displaystyle {\overline {AC}},}$ ergibt Schnittpunkt ${\displaystyle E.}$
5. Ziehe einen Halbkreis um ${\displaystyle D}$ ab ${\displaystyle E}$ im Uhrzeigersinn, ergibt Schnittpunkt ${\displaystyle F.}$
6. Halbiere die Strecke ${\displaystyle {\overline {DE}}}$ in ${\displaystyle G.}$
7. Verbinde ${\displaystyle G}$ mit ${\displaystyle C,}$ ergibt Schnittpunkt ${\displaystyle H.}$
8. Bestimme den Punkt ${\displaystyle J}$ so, dass ${\displaystyle |FJ|={\overline {MC}}={\sqrt {2}}\cdot r.}$
9. Verbinde ${\displaystyle J}$ mit ${\displaystyle H}$ ergibt Schnittpunkt ${\displaystyle K.}$
10. Zeichne eine Parallele zu ${\displaystyle {\overline {BC}}}$ ab ${\displaystyle K}$ bis Strecke ${\displaystyle {\overline {MB}},}$ ergibt Schnittpunkt ${\displaystyle L.}$

Wird die Strecke ${\displaystyle {\overline {KL}}}$ verdoppelt, ergibt dies die Seitenlänge ${\displaystyle a}$ eines Quadrates mit einem Flächeninhalt, der nahezu gleich dem des Kreises ist.

Bei einem Kreis mit Radius r = 1 [LE]:

• Konstruierte Seite des Quadrates a = 1,772453865554221... [LE]
• Soll-Seite des Quadrates a_s = ${\displaystyle {\sqrt {\pi }}\cdot 1[LE]}$ = 1,772453850905516... [LE]
• Absoluter Fehler = a - a_s = 0,000000014648705... = 1,4648...E-8 [LE]
• Fläche des konstruierten Quadrates A = a² = ‭3,141592705518100‬... [FE]
• Soll-Fläche des Quadrates A_s = ${\displaystyle {\pi }\cdot 1[FE]}$ = 3,141592653589793... [FE]
• Absoluter Fehler = A - A_s = 0,000000051928307... = 5,1928...E-8 [FE]
• Fazit: Sieben Nachkommastellen sind gleich denen von ${\displaystyle {\sqrt {\pi }}}$ bzw. sechs Nachkommastellen sind gleich denen von ${\displaystyle \pi }$.
  • Bei einem Kreis mit dem Radius r = 100 km wäre der Fehler der Seite a ≈ 1,5 mm
  • Bei einem Kreis mit dem Radius r = 10 m wäre der Fehler der Fläche A ≈ 5,2 mm²

The approximation of ${\displaystyle {\frac {a}{2}}}$ is based on value

${\displaystyle {\overline {KL}}={\frac {a}{2}}=1-{\frac {{\sqrt {2}}\left(2-{\sqrt {2}}\right)\left(2-{\sqrt {2}}+{\sqrt {{\frac {1}{2}}+{\sqrt {2}}}}\right)}{-11+11{\sqrt {2}}+5{\sqrt {1+2{\sqrt {2}}}}}}\approx r\cdot \ 0.886\;226\;932\;777\;110\;[\mathrm {ul} ]}$

The use of the square root of 2 = ${\displaystyle |FJ|}$ provides an approximation of ${\displaystyle \pi ,}$ which was almost achieved in 1913 in the construction by Jakob de Gelder with the Zu Chongzhi fraction ${\displaystyle {\frac {355}{113}}.}$

• Result of the following construction

${\displaystyle 3.141\;592\;{\color {red}\;705\;\ldots }}$

• Result with Zu Chongzhi fraction ${\displaystyle {\frac {355}{113}}}$

${\displaystyle 3.141\;592\;{\color {red}\;920\;\ldots }}$

• Circle number ${\displaystyle \pi }$

${\displaystyle 3.141\;592\;653\ldots }$

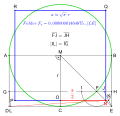
1. Draw a circle with any radius ${\displaystyle r}$ around its center ${\displaystyle M.}$
2. Draw the square ${\displaystyle MBCA}$ with ${\displaystyle r}$ as the side length and extend the line segment ${\displaystyle {\overline {CB}}}$ beyond ${\displaystyle B.}$
3. Draw the diagonal ${\displaystyle {\overline {MC}},}$ results in intersection point ${\displaystyle D.}$
4. Draw a parallel to ${\displaystyle {\overline {BC}}}$ through ${\displaystyle D}$ to ${\displaystyle {\overline {AC}},}$ results in intersection ${\displaystyle E.}$
5. Draw a semicircle around ${\displaystyle D}$ clockwise, results in intersection point${\displaystyle F.}$
6. Halve the line segment ${\displaystyle {\overline {DE}}}$ in ${\displaystyle G.}$
7. Connect ${\displaystyle G}$ to ${\displaystyle C,}$ results in intersection ${\displaystyle H.}$
8. Find the point ${\displaystyle J}$ such that ${\displaystyle |FJ|={\overline {MC}}={\sqrt {2}}\cdot r.}$
9. Connect ${\displaystyle J}$ to ${\displaystyle H,}$ results in intersection point ${\displaystyle K.}$
10. Draw a parallel to ${\displaystyle {\overline {BC}}}$ from ${\displaystyle K}$ to line segment ${\displaystyle {\overline {MB}},}$ results in intersection ${\displaystyle L.}$

If the line segment ${\displaystyle {\overline {KL}}}$ is doubled, this results in the side length ${\displaystyle a}$ of a square with an area that is almost equal to that of the circle.

In a circle of radius r = 1 [unit length, ul]:

• Constructed side of the square a = 1.772453865554221... [${\displaystyle ul}$]
• Target side of the square a_s = ${\displaystyle {\sqrt {\pi }}\cdot 1[ul]}$ = 1.772453850905516... [${\displaystyle ul}$]
• Absolute error = a - a_s = 0.000000014648705... = 1.4648...E-8 [${\displaystyle ul}$]
• Surface of the constructed square A = a² = 3.141592705518100... [unit area, ua]
• Target area of the square A_s = ${\displaystyle {\pi }\cdot 1[ua]}$ = 3.141592653589793... [${\displaystyle ua}$]
• Absolute error = A - A_s = 0.000000051928307... = 5.1928...E-8 [${\displaystyle ua}$]
• Conclusion: seven decimal places are equal to those of ${\displaystyle {\sqrt {\pi }}}$ respectively six decimal places are equal to those of ${\displaystyle \pi }$.
  • In a circle of radius r = 100 km would be the fault of the page a ≈ 1.5 mm
  • In the case of a circle with the radius r = 10 m would be the error of the surface A ≈ 5.2 mm²

Ich, der Urheber dieses Werkes, veröffentliche es unter der folgenden Lizenz:

Diese Datei ist lizenziert unter der Creative-Commons-Lizenz „Namensnennung – Weitergabe unter gleichen Bedingungen 4.0 international“.

Dieses Werk darf von dir

• verbreitet werden – vervielfältigt, verbreitet und öffentlich zugänglich gemacht werden
• neu zusammengestellt werden – abgewandelt und bearbeitet werden

Zu den folgenden Bedingungen:

• Namensnennung – Du musst angemessene Urheber- und Rechteangaben machen, einen Link zur Lizenz beifügen und angeben, ob Änderungen vorgenommen wurden. Diese Angaben dürfen in jeder angemessenen Art und Weise gemacht werden, allerdings nicht so, dass der Eindruck entsteht, der Lizenzgeber unterstütze gerade dich oder deine Nutzung besonders.
• Weitergabe unter gleichen Bedingungen – Wenn du das Material wiedermischst, transformierst oder darauf aufbaust, musst du deine Beiträge unter der gleichen oder einer kompatiblen Lizenz wie das Original verbreiten.

https://creativecommons.org/licenses/by-sa/4.0CC BY-SA 4.0 Creative Commons Attribution-Share Alike 4.0 truetrue